What Even is Trigonometry?

Studying trigonometry at school is a rite of passage. Student’s experience is diverse, and I’d like to think many enjoy it, but some find it challenging. However, they all seem to remember something about it, if only just the name. Ask a student what trigonometry is and you will get some varied responses, such as “Its some buttons on a calculator sin, cos, tan” or “you use it to work stuff out in triangles” or worse some odd (sometimes rude) mnemonic about a Silly Odd Hipo… as if that tells them something about what it is. Often this is successful in that students are able to routinely work with the ratios and derive angles and lengths using them, but do they have any understanding of what trigonometry really is?
I might argue trigonometry is one of the most beautiful parts of the maths course at GCSE and one which should be explored in its full beauty. Its links to ratio, similarity, geometry, and algebraic manipulation make it the summit of the GCSE course. Planning this curriculum journey to the summit requires careful thought and sequencing to ensure students make the most of the views on the way up. Later in this post I provide some thoughts and ideas on how this might be sequenced, scattered through a curriculum.
Where Does Trigonometry Come From?
Documentation on exactly where and who first used the ideas of trigonometry is hard to find. But we do know that, about two thousand years ago, one of the first places trigonometry is clearly seen is when Hipparchus was determining the distance of the of the Sun from the Earth using the Sun’s apparent orbit. Apparent because they thought then that the Sun orbited the Earth.
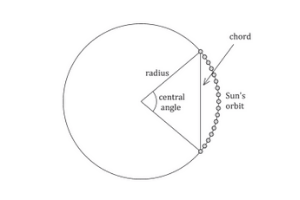
Figure 1
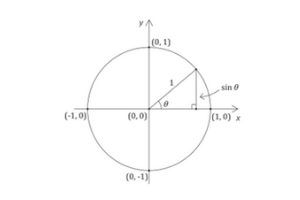
Figure 2
It was through this geometric modelling [Figure 1] that Hipparchus found a relationship between a chord length (the distance from one side of a circle to another) and the radius made by different angles. What he found was by varying the size of the radius at an angle it meant that, due to its similarity, the ratios of chord to radius always remained constant. This was known as the Fundamental Problem of Trigonometry. Two hundred years later Ptolemy then documented systematically a table of these ratio values for each half degree up to 180o and this was recorded as the first table of sines – which was amazingly accurate up to 5/6 decimal places.
These ideas can be better seen when transferred to a coordinate grid to form a unit circle. This unit circle is centred at the origin (0,0) and has radius 1 [Figure 2]. From this image its usefulness is apparent as it contains within it the very essence of trigonometry.
Many might be more familiar with the idea of linking trigonometry to triangles, but trigonometry originates from circles and is also often called the circular functions. Starting at the point (1, 0) and rotating the radius an angle anti-clockwise then the sine of that angle, the height, is now defined as a point on the unit circle [Figure 2].
Sunny Trigonometry
Sunny sine is one way in which I like to think of what sine of an angle is. As the Sun rises from (1,0) we can then track the height of the Sun. The bonus from this understanding is that students can also draw the graph easily as they can visualise the sine(0) = 0, sine(90) = 1 and sine(180) = 0 as the Sun moves across the sky as key points on the path of the graph too.
What is the cosine of an angle? Using the Sun analogy again then we can think of it as the distance the Sun is across from us in the sky for a given rotation. On our coordinate grid this is the x-coordinate.
A beautiful consequence of this is it follows that using Pythagoras’ theorem (sin θ)2 + (cos θ)2 = 1. This is an important trigonometric identify that students need for A-level and now they will be able to recognise its origin. Having this background understanding of the unit circle and how it links to the trigonometric ratios makes this much easier for students to grasp and importantly remember.
Finally, the tangent. Well, this depends on how steep the Sun is in the sky. The tangent of the angle is the length of this tangent segment from its radius to the horizon. Within the resources shared below there are some helpful graphical representations to help visualise this.
Curriculum Sequencing of Trigonometry
With a brief overview of what trigonometry is, you can see how it can be related to many other areas of the curriculum. I know I have been guilty of fobbing off younger students when asked what these strange buttons on their calculator do, by saying something along the lines of … “Oh you’ll learn about that later” or “its big person mathematics wait till year 9”. But there is often curiosity there and maybe the foundations can be laid, and seeds can be planted earlier with careful sequencing and planning for a better understanding of trigonometry.
There are many ways to the summit of trigonometry at GCSE. Each of these routes maybe different depending on the students you have and their prior learning and many of these different routes have pros and cons to them. For example, knowing when to teach the ‘why’ in trigonometry is a difficult choice. Ensuring that students are not overloaded too early with such a new topic can affect their motivation. Therefore, approaching trigonometry across the years and spacing the learning may have many benefits to reduce the cognitive load and develop a deeper understanding of what trigonometry is.
What follows is just my rough progression of ideas of how this might be spaced across years with some related tasks on how to incorporate the unit circle into the teaching of trigonometry.
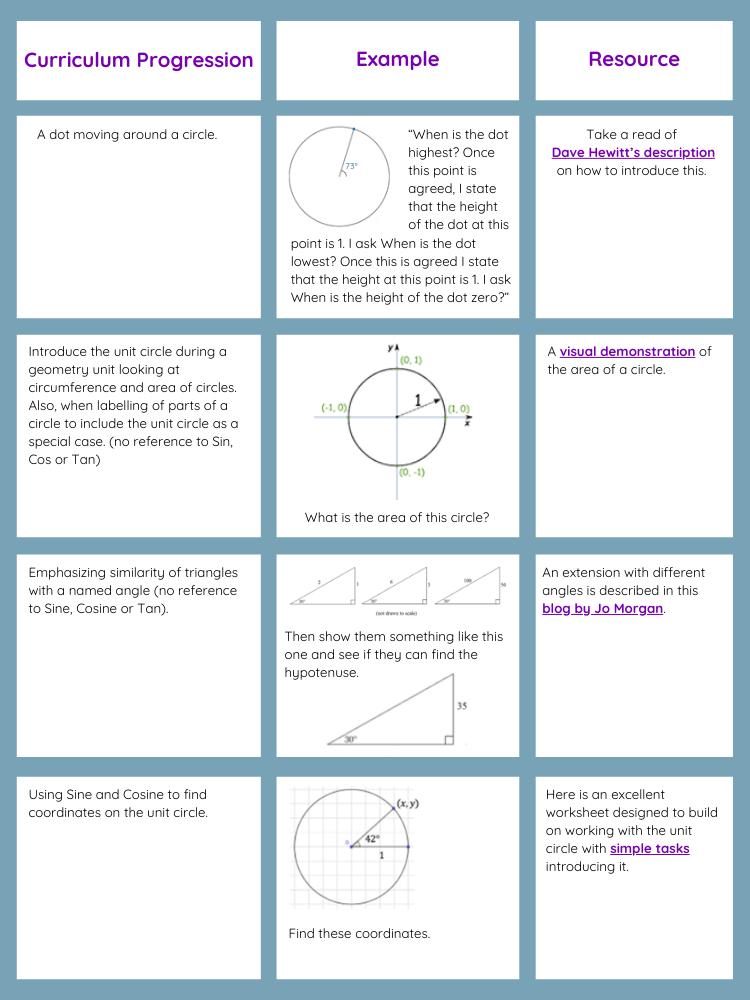
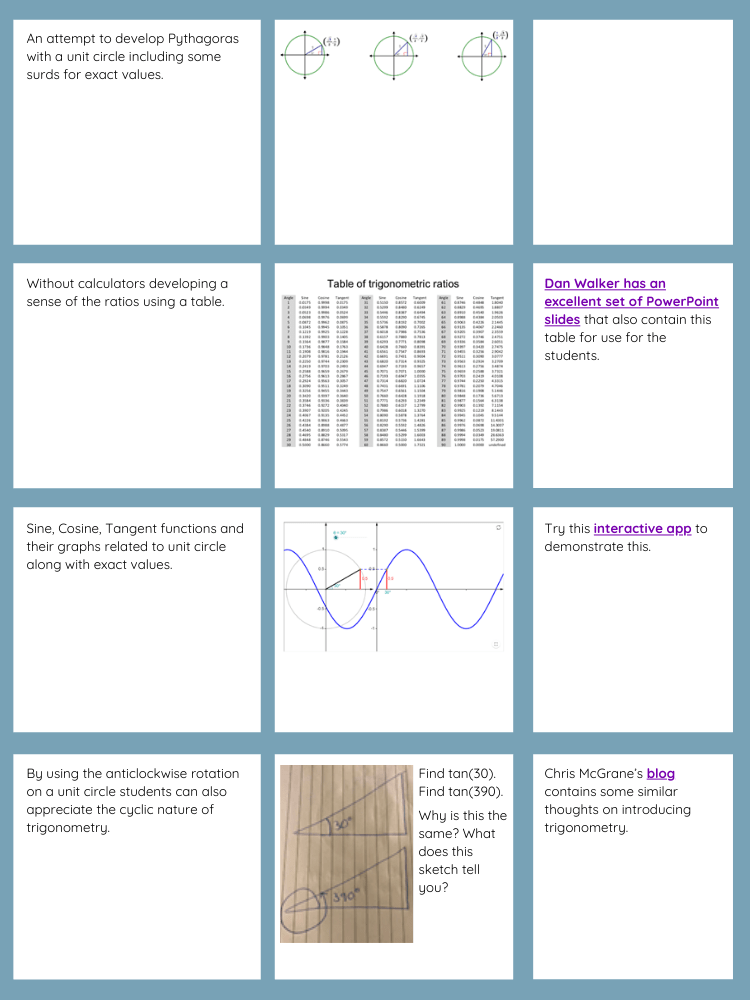
Summary
In summary, trigonometry was once used to help us find the distances between the Earth and the Sun and as a result helped formulate the relationships of angles and radius to the lengths of chords. This application connects the circle to the triangle, and to the graphs as waves. It is a beautiful topic with a history that can also help understanding what trigonometry is. I invite you to take the challenge and attempt teaching this topic with its original meaning.


